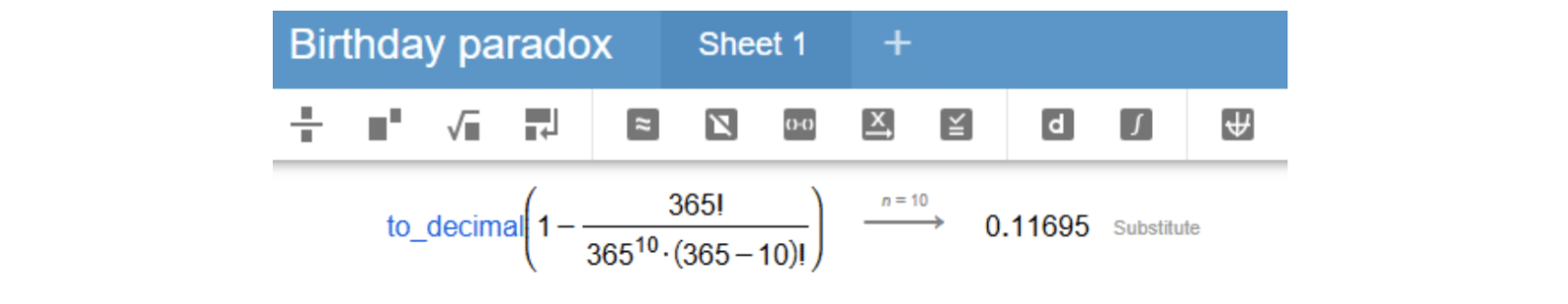
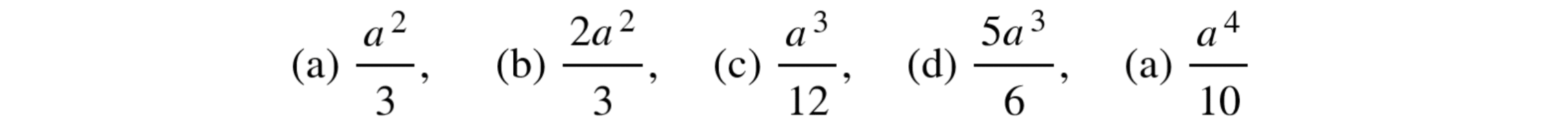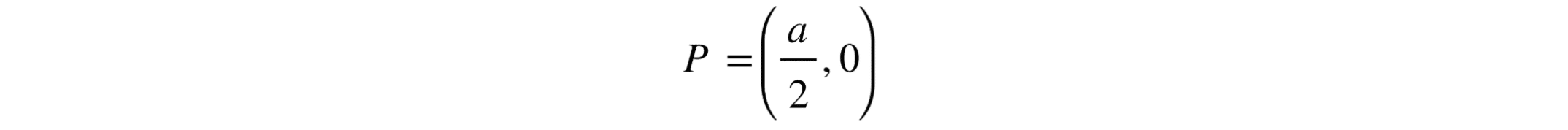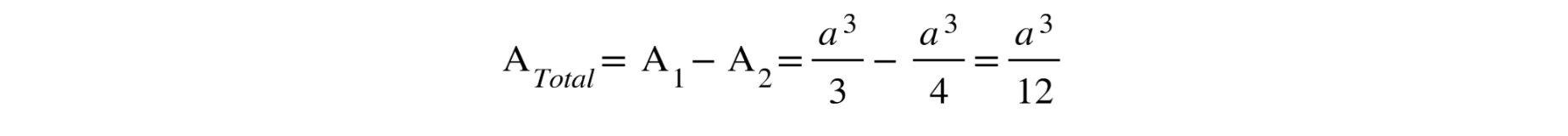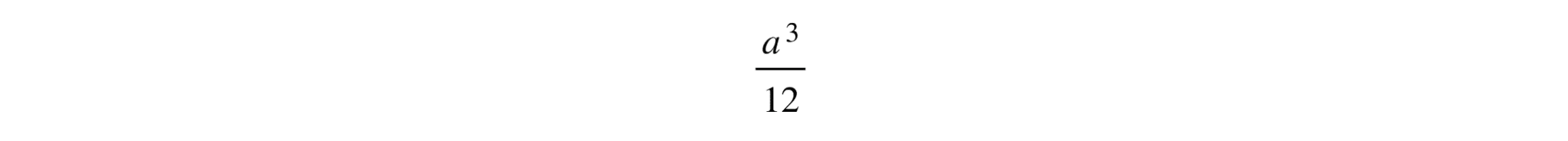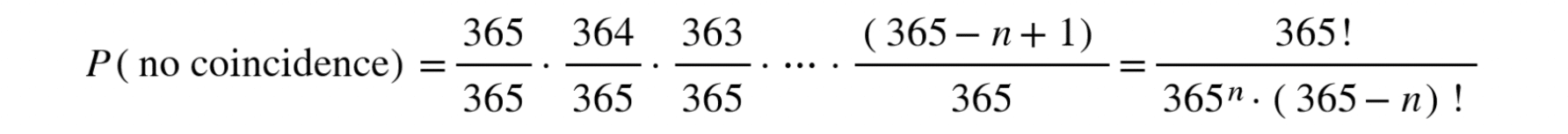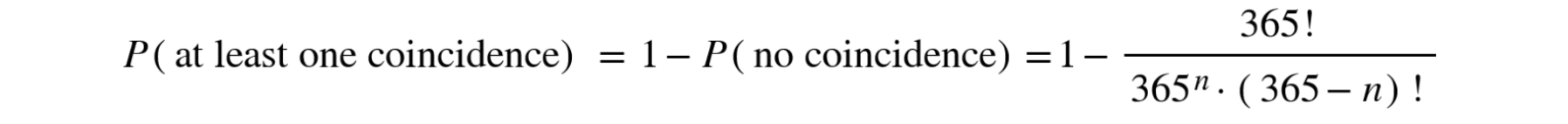Post type: Industry

Hybrid Learning in Math Education: Why Blending Online and Offline Instruction Matters

Post author By Aida Alsina
Post date September 9, 2025

Hybrid learning, also known as blended learning, is an educational approach that combines traditional face-to-face instruction with digital, online components. Instead of treating online and offline learning as separate modes, hybrid learning integrates both in a coordinated and intentional way. The goal is not simply to alternate between classroom and remote activities, but to design a cohesive experience that leverages the strengths of each environment—flexibility, accessibility, interaction, and personal connection. This model has gained significant traction in recent years, especially in math education, where abstract concepts and diverse learning needs benefit from varied modes of engagement.

Hybrid learning is reshaping how we teach and learn math. By blending digital tools with in-person instruction, educators can offer more personalized, effective, and engaging learning experiences.

This approach doesn’t replace traditional methods: it enhances them. It respects the emotional and cognitive aspects of learning and responds to the reality of modern classrooms, where students have diverse learning paces, styles, and circumstances.

Blended learning is not a trend but a pedagogical shift that bridges accessibility, adaptability, and human connection.

The Power of Combining Online and Offline Math Instruction

Blended learning in math education creates a synergy that neither modality can achieve alone. As math educators adapt to evolving student needs and technological possibilities, this combined model offers:

Flexibility: Students can access digital resources anytime, which is particularly helpful for review or reinforcement outside the classroom.
Interactivity: Online tools bring math concepts to life through animations, simulations, and interactive problem-solving platforms.
Human Connection: Face-to-face instruction fosters real-time discussion, emotional support, and immediate feedback.
Scalability: Educators can manage large or diverse groups more efficiently, offering tailored support where needed.

In math, where abstract concepts often require iterative exploration, hybrid learning empowers both guided instruction and independent thinking. It accommodates repetition, variation, and collaborative exchange, all crucial for deep understanding. By combining the immediacy of in-person learning with the accessibility of online math teaching, educators can truly transform their practice.

Balance Between Structure and Autonomy in Hybrid Learning

One of the central strengths of hybrid learning is its ability to balance learning framework and autonomy. Math learning demands both: structured pathways to introduce and build skills progressively, and autonomy to foster individual exploration, confidence, and critical thinking. Blended learning strategies provide the framework for this balance, supporting both consistency and creativity in math classrooms.

Online: Providing Structure and Individual Pacing

Online environments offer a structured framework for learning. Through curated modules, step-by-step explanations, and automated feedback, students gain clarity and consistency. The self-paced nature of online platforms allows students to revisit concepts, practice at their own rhythm, and receive immediate guidance, which is especially valuable for mastering foundational skills.

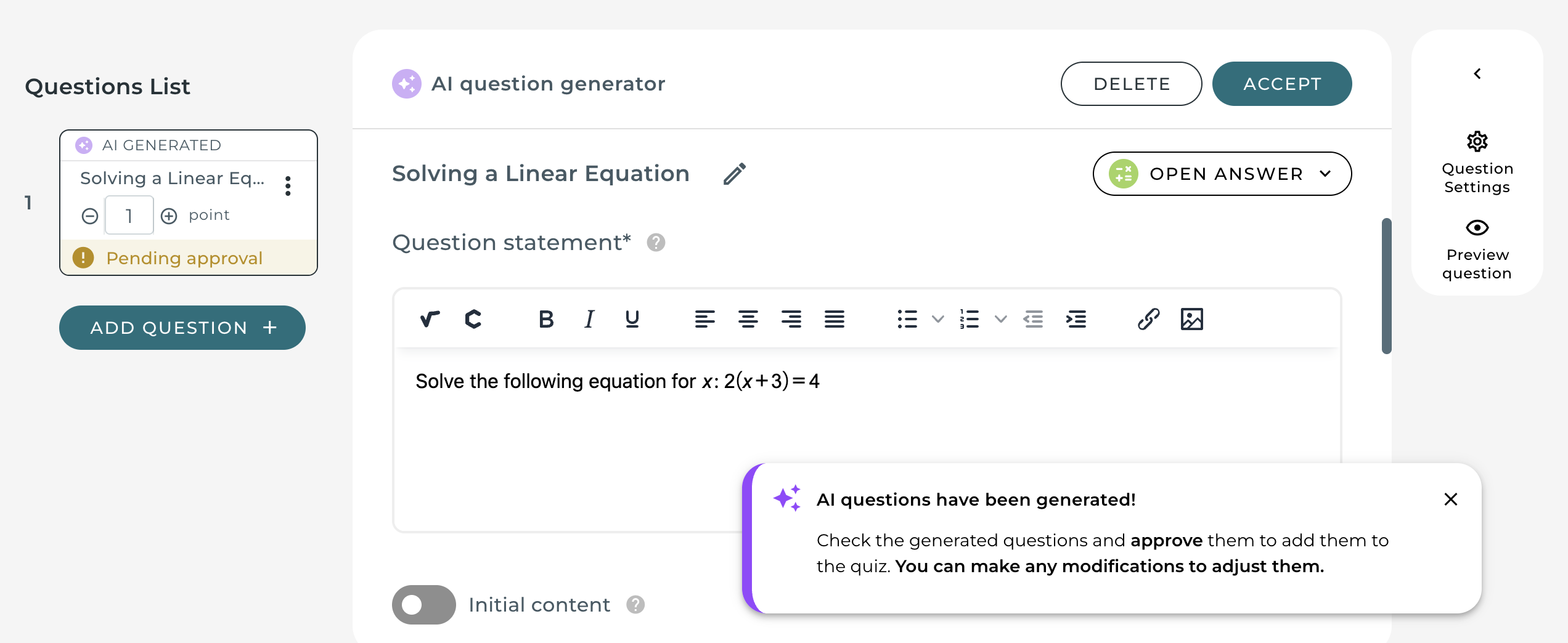
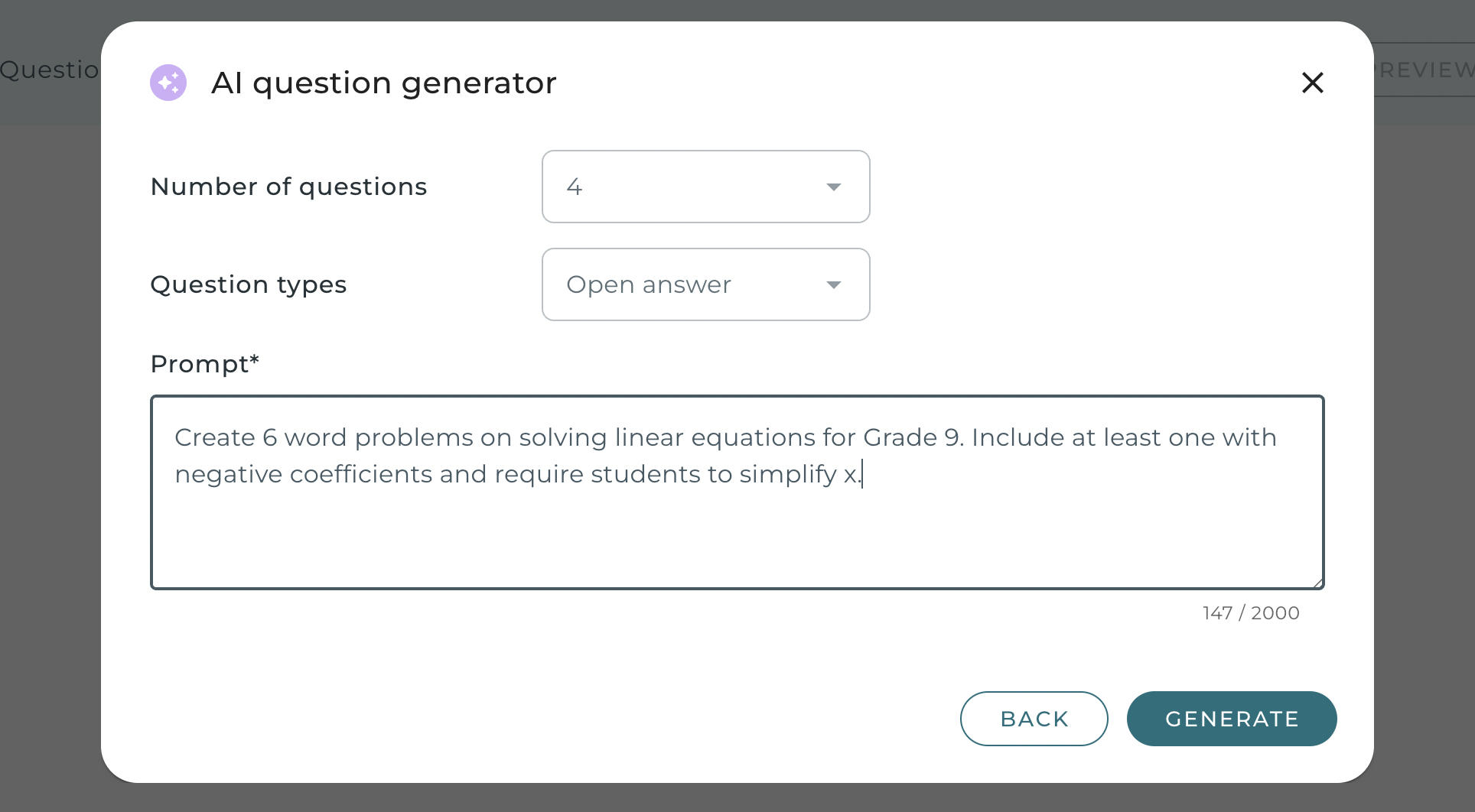
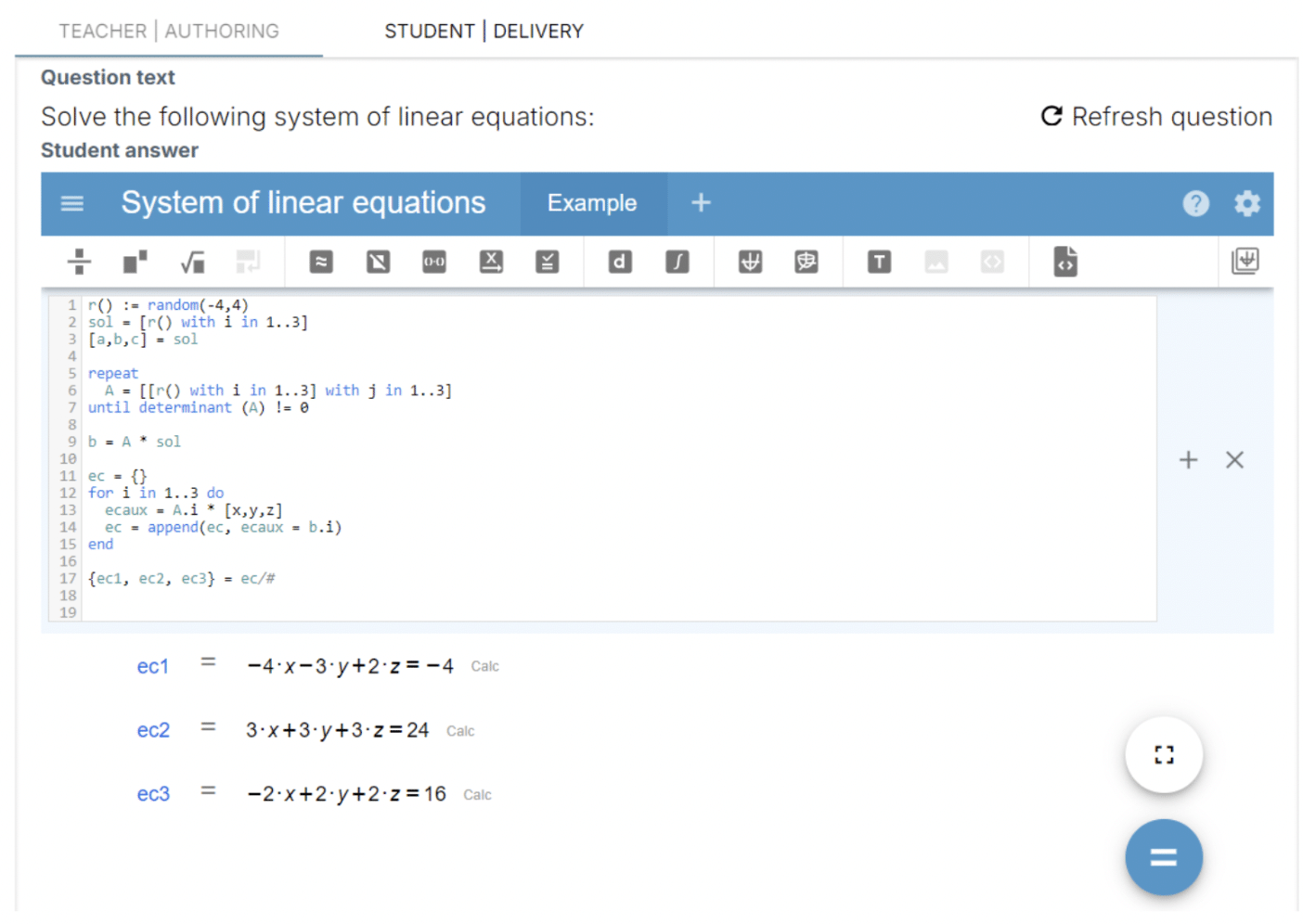
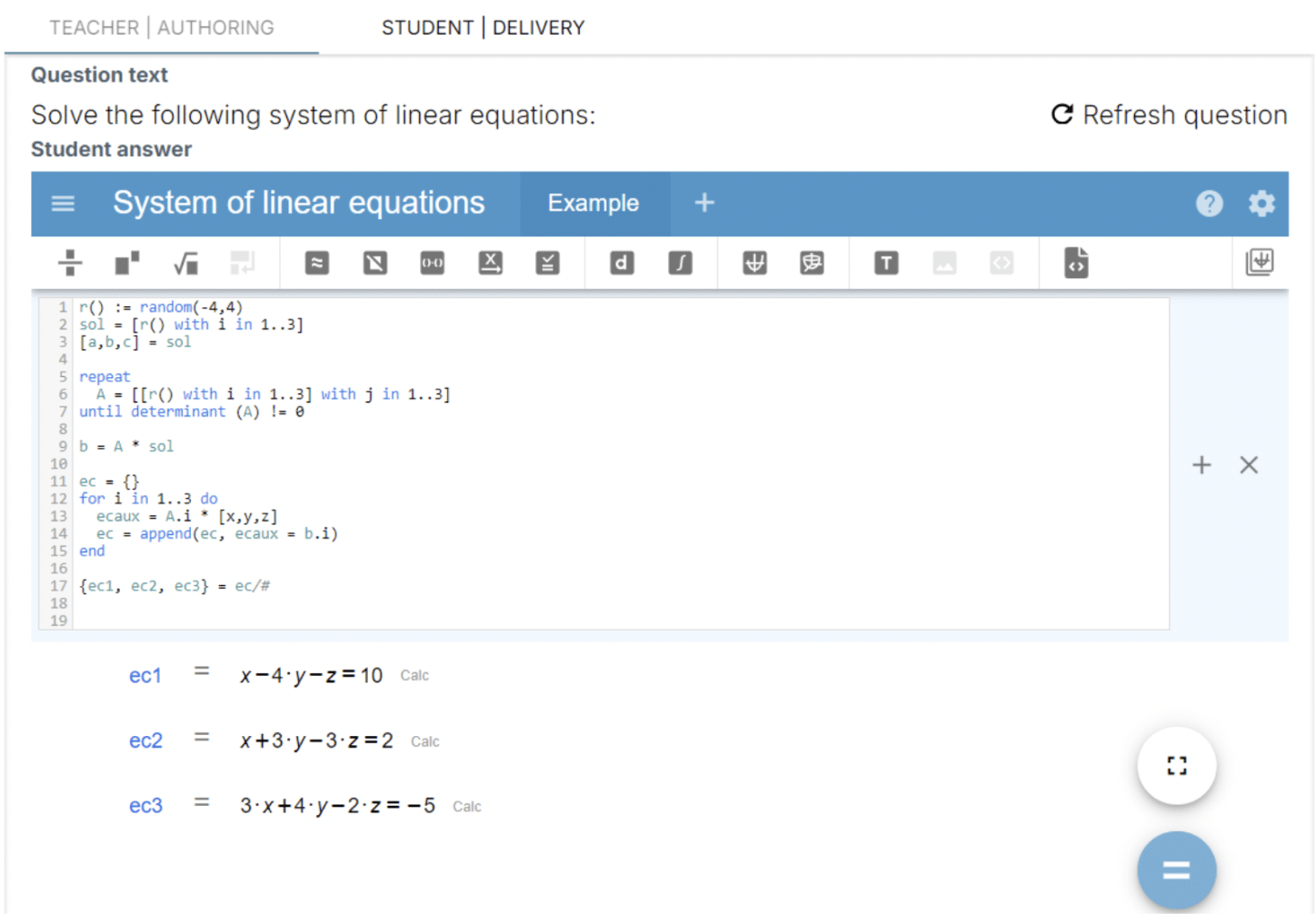
Tools like WirisQuizzes, a platform designed specifically for math and STEM assessments, enhance this experience by offering dynamic, auto-corrected questions with random variables. This not only provides individualized practice opportunities but also ensures that students engage actively with the material rather than passively memorizing answers. Online math teaching empowers learners by offering them control over their pace and focus areas, reinforcing competence and motivation.

Offline: Promoting Dialogue and Deeper Reasoning

Offline, classroom-based instruction adds the relational and spontaneous aspects of learning. In-person sessions encourage students to articulate their reasoning, ask questions, and collaborate on complex problems. Teachers can detect misunderstandings in real time and adapt explanations. These interactions develop mathematical communication skills and help students connect theory with practice. This live component of hybrid learning ensures that math remains a social, responsive, and human-centered discipline.

Together, these modalities reinforce each other. Structure without autonomy may feel rigid; autonomy without structure may lack direction. Hybrid learning aligns both.

Visual and Symbolic Thinking

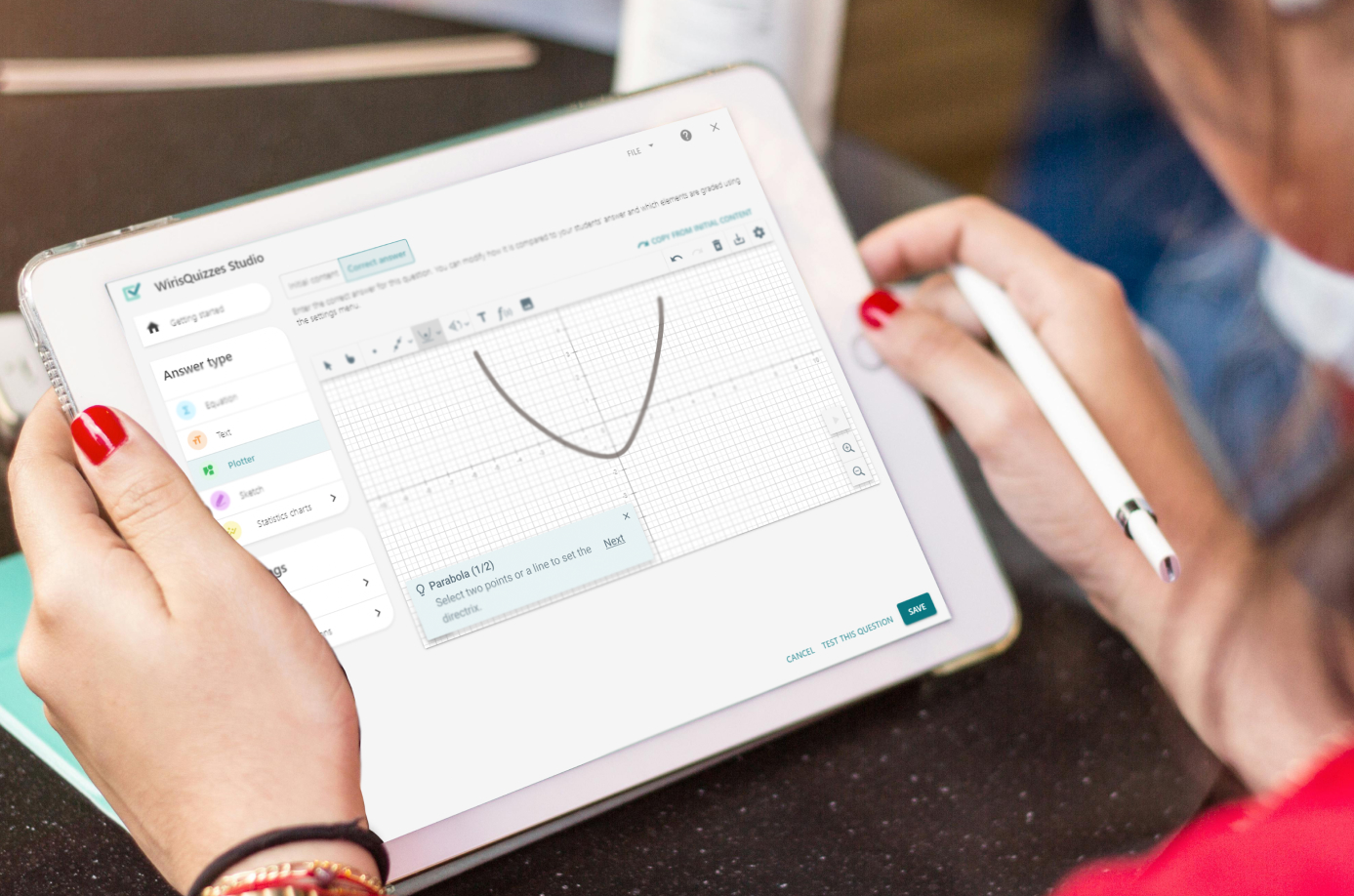
Math is both visual and symbolic. Hybrid learning supports this duality by using digital tools to represent abstract ideas and classroom environments to interpret and discuss them.

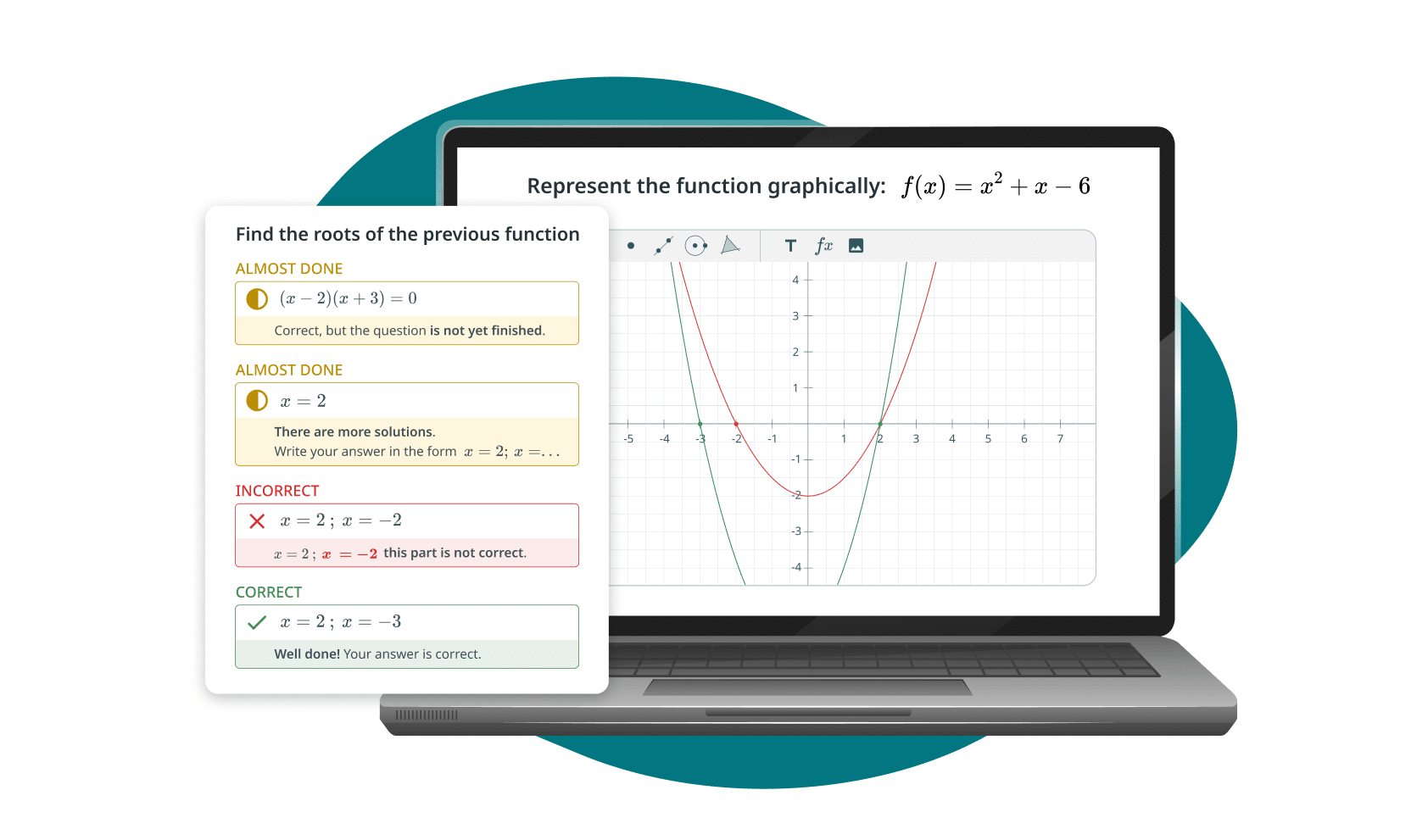
Online platforms like WirisQuizzes or Learning Lemur (our intuitive math platform integrated with Google Classroom that provides customizable quizzes, automatic grading, and personalized feedback) can visualize functions, geometric transformations, or algebraic manipulations interactively. These representations complement traditional symbolic work and help learners form mental models. In-person settings then provide the space to reflect on and interpret these representations with peers and educators, solidifying understanding.

school learning

By moving fluidly between visualizations online and symbolic manipulation offline (and vice versa), students strengthen their conceptual grasp. This kind of dynamic interplay is a key benefit of hybrid learning, enabling learners to connect different forms of mathematical representation more effectively.

Real-World Applications of Hybrid Learning in Math

Hybrid learning is not just a theoretical framework, it is being put into practice across a wide variety of educational settings. From urban schools to rural learning centers, educators are designing innovative ways to integrate digital and face-to-face instruction in their math classrooms. These practical applications demonstrate how blended learning strategies can meet diverse educational needs while maintaining quality and engagement.

Among the many institutions that already apply the hybrid learning system, we can find 21K School (United Kingdom), Harvard University (USA), Massachusetts Institute of Technology (USA), and public schools in Singapur.

Use Cases from Classrooms Worldwide

A high school teacher flips the classroom: students watch instructional videos and complete guided exercises at home, while class time is used for collaborative problem-solving.
A university professor alternates between digital assessments and in-person workshops, combining online scalability with live, hands-on support.
A community center in a low-connectivity area uses downloaded interactive materials during the week and organizes weekend math clubs with volunteer educators.

These real-world models show how hybrid learning can be tailored to different contexts, always seeking to optimize both access and engagement.

A Human-Centered Vision of Technology: The Wiris Approach

Wiris strives to maintain a modern and technological outlook without losing sight of the human and natural essence of education and scientific inquiry. In Wiris, we believe that true innovation does not come at the expense of personal connection, but it builds upon it. This philosophy aligns perfectly with the hybrid learning model, which combines the strengths of digital tools with the irreplaceable value of in-person instruction.

Wiris designs tools that reflect this vision. These tools are not about replacing educators or standardizing learning. They aim to empower teachers and students within the hybrid model that embraces this balance of structure and autonomy, visual and symbolic reasoning, digital reach, and human connection.

WirisQuizzes, for example, supports hybrid education with customizable, automatically graded math quizzes powered by random variables—ensuring individualized learning while maintaining academic rigor.

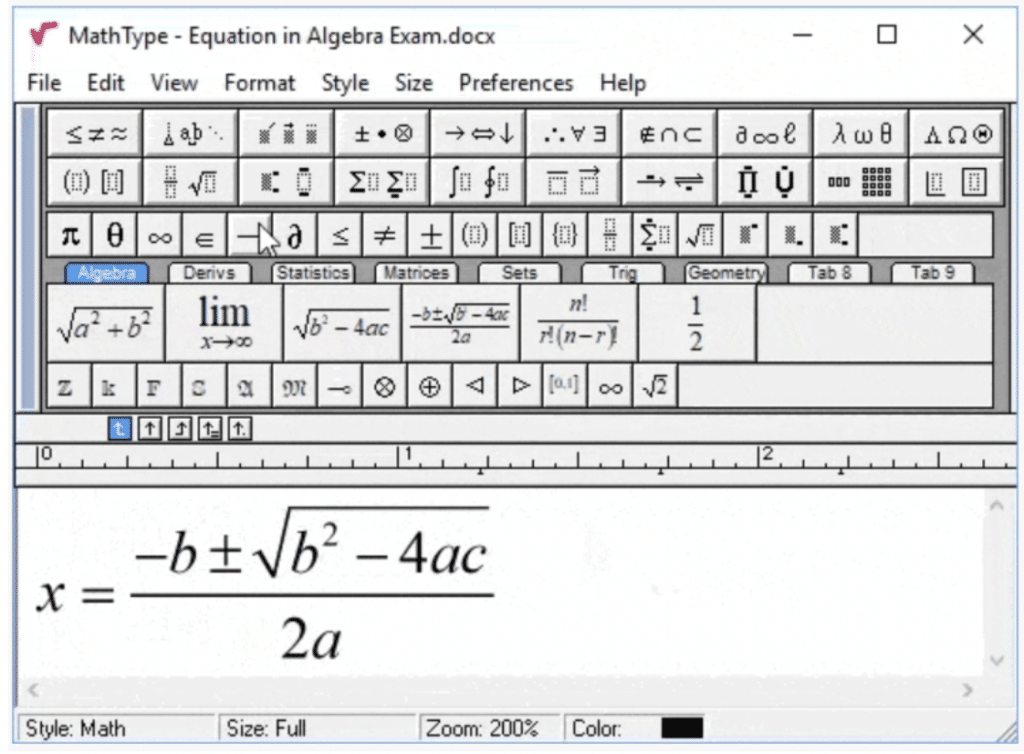
MathType complements this approach by providing an intuitive editor for writing mathematical notation across multiple platforms, enabling seamless symbolic communication in both digital and physical learning environments.

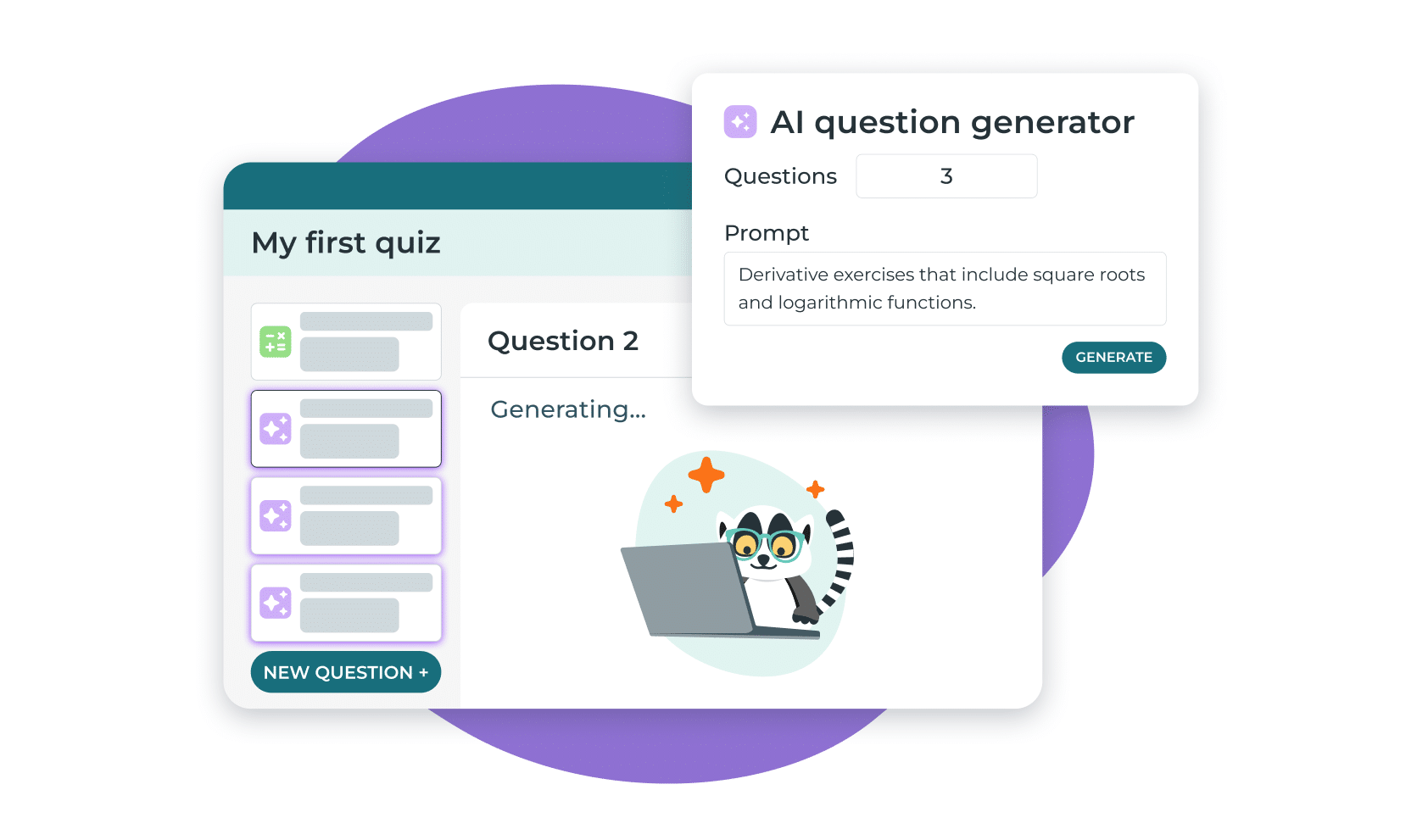
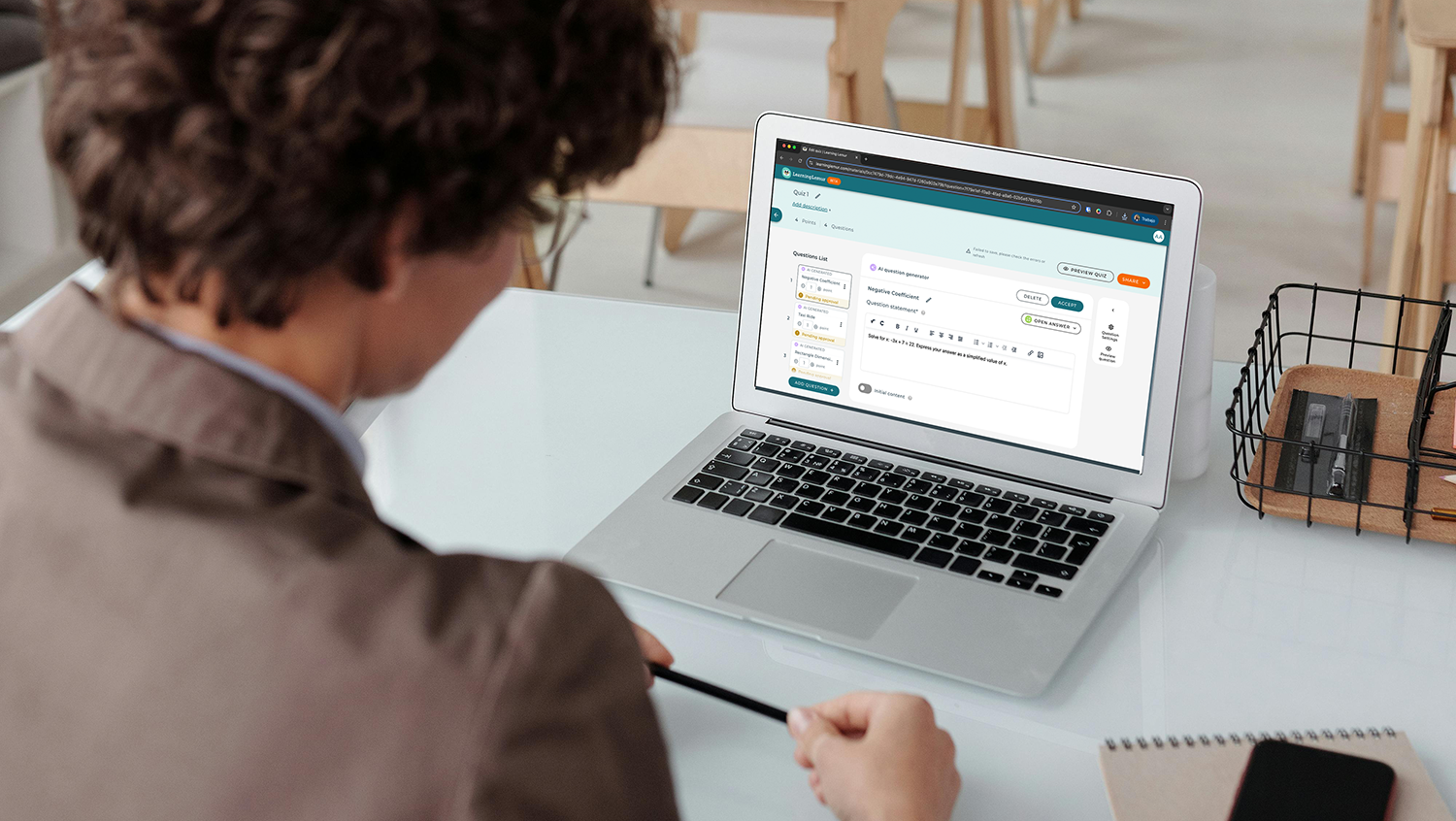
LearningLemur, our intuitive math platform designed specifically for Google Classroom, offers interactive, customizable quizzes with automatic grading and personalized feedback. It transforms how students engage with math exercises, providing visual support and adaptable content that fits any hybrid learning strategy.

Embracing the Future of Math Instruction

As the line between digital and physical classrooms continues to blur, embracing hybrid learning isn’t just an option: it’s a necessity. With the right methodology and the right tools, we can build a future of math instruction that is inclusive, adaptive, and deeply connected to the needs of students and educators alike.

Explore how Wiris supports hybrid learning here

Uncategorized

From Education to Engineering: Why Efficient Math Writing Matters

Post author By Aida Alsina
Post date August 18, 2025

In today’s data-driven world, math content has become a core asset for innovation, communication, and decision-making. Whether you’re designing algorithms, explaining a financial model, or building educational materials, math writing plays a key role in turning complex ideas into actionable insights.

Creating accurate, clear, and accessible math content is no longer a task limited to academia: it is a necessity for sectors that rely on precision and knowledge transfer.

Where Math Content Creation Matters Most

Numerous industries and knowledge domains depend on the ability to produce, adapt, and distribute high-quality math content:

Education and EdTech

In the educational ecosystem, math content creation is foundational to the learning experience. Teachers, curriculum designers, edtech developers, and instructional designers rely heavily on precise math writing to prepare lesson plans, assessments, and interactive digital resources. From primary education to advanced university-level courses, the clarity of mathematical expressions can directly impact student comprehension and performance.

Moreover, in EdTech platforms, the challenge is not only pedagogical but also technological: math equations must be compatible with multiple devices, formats, and learning management systems (LMS). An efficient math editor empowers educators to quickly create, edit, and share content that maintains its structure and meaning across platforms. In this context, robust math content creation tools are essential to promote equity, consistency, and accessibility in modern education.

mathtype editor — *Example of math formula written with Wiris’ MathType*

Scientific Communication and Research

Research in fields like physics, chemistry, biology, and mathematics relies on formalized, structured math writing to present hypotheses, validate results, and communicate findings. From peer-reviewed journals to preprints and grant applications, the presentation of math content can influence not only understanding but also the perceived credibility and professionalism of the work.

In scientific communication, reproducibility is key, and that begins with clear, accurate notation. A powerful math editor allows researchers to format complex expressions with ease, whether writing in LaTeX or using WYSIWYG interfaces. Additionally, the ability to produce accessible and well-structured math content supports broader dissemination and interdisciplinary collaboration, enabling scientists to reach audiences beyond their immediate field.

Engineering and Technical Fields

Engineers and technical professionals deal with complex models, formulas and simulations daily. Whether it’s civil, mechanical, electrical or software engineering, math writing is central to documenting calculations, designing systems and validating outcomes. A minor error in notation can lead to misunderstandings or costly consequences in design and implementation.

Creating high-quality math content in this context requires precision, standardization, and compatibility with technical documentation tools. An advanced math editor enables teams to present formulas clearly, integrate them into CAD documentation or specifications, and ensure that the mathematical logic behind a project is both transparent and reproducible. In collaborative engineering environments, clarity in math content helps avoid ambiguity and streamlines review and approval processes.

Economics and Finance

In economics and financial analysis, mathematics is the language of logic and prediction. Economists, analysts, and consultants must build models, define formulas, and perform complex calculations that underpin reports, forecasts, and strategic decisions. The credibility of these outputs often depends not just on the numbers but on how clearly the underlying methodology is communicated.

Effective math writing is crucial to ensure that stakeholders -be they clients, regulators, or internal teams- understand and trust the content being presented. A professional math editor facilitates the creation of visually coherent, publication-ready equations that can be embedded in reports, dashboards or academic publications. By investing in better math content practices, financial institutions and think tanks can enhance transparency and drive data-informed decisions.

Corporate Innovation and Data Teams

In corporate environments, especially within innovation, R&D, and data science teams, math content is integral to documenting algorithms, models, and analytical frameworks. These teams often work cross-functionally with product managers, developers, and decision-makers, making it essential to express complex logic in a format that is both technically correct and easily interpretable.

Whether it’s machine learning formulas, operational models, or optimization strategies, professional math writing improves internal communication and project documentation. A reliable math editor can help standardize the way mathematical reasoning is presented across teams and documents, accelerating onboarding, compliance, and strategic planning. As companies increasingly rely on data-driven methodologies, structured math content becomes a competitive advantage.

The Value of Clear and Consistent Math Writing

High-quality math writing opens the door to significant strategic advantages for any organization. When math content is clear, accessible, and professionally formatted:

Technical data is interpreted correctly, enabling informed decision-making across teams and stakeholders.
Research and analysis are communicated clearly, enhancing dissemination, credibility, and engagement with diverse audiences.
Efficiency improves, as well-formatted math content reduces the need for time-consuming corrections and rework.
Cross-functional collaboration becomes easier since standardized content facilitates understanding between departments, institutions, or international partners.

By prioritizing structured and coherent math content, organizations position themselves for success in education, research, development, and beyond.

MathType: A Math Editor Designed for Today’s Needs

Once we acknowledge the strategic value of math content, the next step is choosing the right tool to support it. MathType is the world’s leading equation editor that allows you to write math notation as easily as you write text. Trusted by educators, researchers and professionals across the globe, it is used by K-12 and university STEM students and faculty worldwide.

Here’s why:

Seamless math writing experience.
MathType enables professionals to write equations as intuitively as regular text. Whether using a keyboard, mouse, or stylus, it adapts to your workflow.

500+ mathematical symbols and structures
MathType offers an extensive symbol library covering arithmetic, algebra, calculus, logic, and more. This allows users to express even the most complex mathematical concepts accurately, avoiding improvisations or workarounds that compromise clarity.
AI-powered handwriting recognition
Users can handwrite equations using a stylus or touch interface, and MathType will instantly convert them into digital math expressions. This accelerates math writing and bridges the gap between traditional note-taking and digital editing.
Export in high-quality formats: SVG, PNG, PDF
MathType generates visually clean equations that can be exported in multiple file formats. Whether for web, print, or presentation use, the quality remains intact, making your math content consistent across platforms.
Accessibility-compliant outputs
The editor ensures all math expressions meet accessibility standards, supporting screen readers and enabling inclusive communication. This is crucial for educational institutions and public sector projects with accessibility requirements.
Integration and customization
MathType integrates with LMS, CMS, HTML/XML editors, and supports customizable formats, making it ideal for scalable projects and diverse user needs.
Support for LaTeX and MathML
Ensure compatibility with standard math markup languages used in academic, technical, and publishing environments.
Includes ChemType for chemistry notation
Edit and format chemical equations with a dedicated toolbar for editing inorganic chemistry notation, supporting scientific professionals with highly specific formatting needs.

editor chemtype — *Example of chemical formula written with Wiris’ MathType*

Invest in Smarter Math Content Creation

In a world where precision, clarity, and accessibility are essential, organizations can no longer treat math writing as an afterthought. Whether you’re educating future scientists, validating engineering designs, or presenting complex financial models, the quality of your math content directly impacts how your message is understood, trusted, and acted upon.

Adopting a professional-grade math editor is not just a matter of convenience: it’s a strategic decision that supports consistency, collaboration, and efficiency across the organization.

Catch up on efficient math equations with MathType

Uncategorized

Teaching Math Online: Transforming Education with Wiris

Post author By Aida Alsina
Post date July 18, 2025

Teaching math online is redefining the educational landscape. The emergence of advanced digital tools has empowered educators to deliver personalized, flexible learning experiences. Among these innovations, innovative online math teaching methods are crucial in enhancing student engagement, knowledge retention, and academic performance. This article explores these transformative strategies and highlights how Wiris enables educators to implement them effectively and efficiently.

Key Trends in Online Math Teaching: Prioritizing Personalization, Engagement, and Flexibility

In an increasingly digital and dynamic educational landscape, modern online math teaching strategies are evolving to address the diverse needs of today’s learners. The focus is no longer solely on content delivery; it has shifted towards creating learning environments that foster personalization, maximize student engagement, and offer flexibility to accommodate different learning styles and paces.

The most prominent and impactful trends in teaching math online today include:

Flipped classrooms: The flipped classroom model transforms the traditional educational paradigm by moving direct instruction outside of the classroom -typically through videos, readings, or online content- and reserving classroom time for active learning. This approach fosters deeper understanding through discussions, collaborative problem-solving, and interactive exercises in math education. Students engage with material at their own pace before class and apply their knowledge during in-person or synchronous online sessions.
Microlearning: Microlearning involves delivering information in small, focused segments that are easier to digest and retain. Rather than overwhelming students with lengthy, complex lessons, microlearning breaks content into concise, manageable units. In innovative online math teaching, this method proves highly effective, as it reduces cognitive overload and enables students to achieve mastery one concept at a time.
Personalized learning: Personalized learning leverages technology to tailor educational experiences to the unique needs, skills, and interests of each student. In the context of online math teaching, this approach allows educators to adapt instruction dynamically, enabling students to progress at their own pace. It ensures that learners receive the right level of support and challenge, enhancing motivation and academic performance.

Wiris solutions, including WirisQuizzes and LearningLemur, represent online tools for teaching math online that operationalize these methodologies, enabling educators to develop dynamic assessments and tailored learning paths for their students.

Real-World Applications: How These Online Math Teaching Strategies Benefit Students

Adopting personalized learning, microlearning and flipped classroom strategies equips students with competencies critical for real-world success. These methodologies not only boost academic outcomes but also nurture skills crucial for thriving in digital and collaborative professional environments.

Through innovative online math teaching methods, educators are fostering the development of vital skills such as problem-solving, critical thinking, and self-directed learning, which are capabilities increasingly demanded in STEM industries and the future workforce.

Flipped Classrooms: Learning by Doing Instead of Memorizing

The flipped classroom model fosters student autonomy by shifting content delivery outside the classroom and reserving in-person sessions for collaborative, higher-order learning activities. Instead of passively listening to lectures, students engage with preparatory materials —such as videos or readings— at their own pace and use classroom time to apply concepts through problem-solving and discussion.

This method promotes critical thinking, deeper understanding, and active participation. It also supports diverse learning styles and encourages students to take greater responsibility for their learning. By transforming the classroom into a dynamic, interactive environment, the flipped model builds key competencies such as communication, collaboration, and analytical skills.

Benefits of the Flipped Classroom Model

These are the main benefits of the Flipped Classroom Model, a teaching approach that is increasingly gaining traction in modern educational methods:

Assign dynamic, preparatory quizzes to prime students for in-class activities. Assigning dynamic, preparatory quizzes ensures students engage with key concepts before class. This preparation allows for more effective in-class sessions focused on applying knowledge rather than covering basics. Tools like WirisQuizzes and LearningLemur can support you in developing these dynamic preparatory quizzes tailored to your learners’ needs.
Foster interactive, discussion-based classroom environments. With prior exposure to the material, class time can be devoted to discussions and collaborative problem-solving. This shift enhances critical thinking and promotes deeper understanding through active dialogue.
Promote active participation and collaborative learning dynamics. Flipped classrooms encourage students to actively participate and collaborate with peers. Working in teams and engaging in problem-solving activities builds not only academic skills but also essential competencies like communication and teamwork.

girl studing online

The Power of Microlearning: Quick Lessons for Better Retention

Microlearning is gaining traction as an effective strategy in online math teaching. This method decomposes complex concepts into manageable units, promoting deeper understanding and better long-term retention.

With WirisQuizzes and LearningLemur, educators can design brief, targeted quizzes that reinforce core concepts efficiently. The immediate feedback mechanisms facilitate real-time learning corrections. These micro-assessments exemplify innovative online math teaching approaches that reduce cognitive load and improve learning efficacy. This tool streamlines microlearning by enabling the creation of interactive, goal-oriented quizzes.

WirisQuizzes incorporates a feature that enhances microlearning by providing tailored feedback for open-ended math questions. It supports three main types of feedback: suggestive feedback, which guides students toward discovering solutions independently; confirmatory feedback, which reinforces correct answers and boosts confidence; and corrective feedback, which identifies mistakes and provides the correct solution. This immediate, adaptive feedback fosters critical thinking and ensures efficient, focused learning, aligning perfectly with microlearning principles.

feedback with wirisquizzes

Personalized Learning: Delivering Tailored Educational Experiences

Personalized learning is a cornerstone of modern educational methodologies, particularly in teaching math online. It leverages technology to customize educational experiences according to the unique needs, skills, and learning speeds of individual students.

WirisQuizzes and LearningLemur are central to enabling this level of personalization:

WirisQuizzes, oriented to higher education, allows teachers to design sophisticated quizzes incorporating mathematical expressions, graphical representations and dynamic text-based responses. Its advanced randomization and evaluation capabilities generate unique assessments for the students, promoting practice through varied and individualized problem sets.
LearningLemur, oriented to K-12 students and seamlessly integrated with Google Classroom, offers a comprehensive platform for customizable quizzes, automated grading, and personalized feedback. It not only saves educators valuable time but also fosters student engagement and mastery. The platform’s extensive library of adaptable exercises and detailed analytics empowers educators to monitor student progress and intervene when necessary, further enhancing personalized learning. In addition, LearningLemur now incorporates an AI math question generator that understands your needs and generates ready-made quizzes in seconds. It’s designed to address one of the most common pain points for educators: how to start creating math assessments efficiently without sacrificing quality.

learning lemur ai questions

What truly differentiates Wiris’ tools from others is their capacity to mathematically interpret any answer, apply rigorous mathematical logic to personalize feedback, and define random variables using advanced algorithms. These capabilities empower educators to offer more precise and responsive learning experiences, ensuring that each student progresses at their optimal pace and receives support tailored to their specific learning trajectory.

By integrating these solutions, educators can create adaptive learning environments where technology dynamically adjusts to student needs, providing instant feedback and continuously aligning educational content with individual learning goals.

Embracing the Future of Education with Wiris

The digital transformation of education demands agile, student-centric teaching methodologies. Teaching math online requires sophisticated tools, and Wiris stands at the forefront, delivering solutions that enable educators to design personalized, interactive learning experiences.

Enhance your online teaching strategy: start using WirisQuizzes today and experience the benefits of next-generation online tools for teaching math online.

Try WirisQuizzes now

Uncategorized

AI for Math: The Smart Way to Create Quizzes Faster

Post author By Aida Alsina
Post date June 12, 2025

Creating engaging and varied math quizzes can be a surprisingly time-consuming task. Teachers often face the dreaded blank page, struggling to come up with fresh problems that match different levels, topics, and student needs. But that’s about to change. With AI for math making its way into classrooms, quiz creation just got a powerful upgrade.

Meet LearningLemur: Your AI Question Generator

LearningLemur is an intuitive math platform integrated with Google Classroom that provides customizable quizzes, automatic grading, and personalized feedback, saving educators time and enhancing student learning. Its extensive library of customizable quizzes and exercises fosters engagement and mastery, while detailed analytics help educators track progress and identify areas for improvement. From simplifying assignment creation to delivering instant feedback, LearningLemur transforms classrooms into dynamic and interactive learning spaces, making math more accessible and engaging for all students.

Now, Wiris has introduced a new feature within LearningLemur: a math question generator that understands your needs and generates ready-made quizzes in seconds. It’s designed to address one of the most common pain points for educators: how to start creating math assessments efficiently without sacrificing quality.

Here’s what LearningLemur’s new AI-powered tool offers:

Describe the type of questions you want.
Receive an instant set of customized math problems.
Edit, refine, and assign them directly to your students.

Forget about starting from scratch. This tool helps you work smarter, not harder, all within a platform that is intuitive and easy to navigate, even for teachers with limited tech experience.

How does it work?

Using this tool is simple and straightforward. Teachers input the number of questions, select the question type (e.g., open answer, multiple choice) and finally provide a prompt (e.g., “Make fractions sum problems for students of grade 12. Ask them to be simplified.”).

The AI handles the rest, offering draft content that aligns with your instructions. And because it’s powered by the trusted Wiris correction engine, you maintain full confidence in the mathematical accuracy.

Why This AI Feature Matters for Educators

The LearningLemur AI question generator isn’t just about saving time. It’s designed to help teachers focus on what really matters: pedagogy, clarity, and curriculum alignment. While the AI handles the generation, teachers can shape the output by ensuring pedagogical alignment with curricular goals, adjusting the complexity and style of each question, and refining the language and clarity for the specific group of learners. This tool does not replace your expertise but accelerates your workflow, allowing you to dedicate more time to effective teaching.

Tips for Writing Effective Prompts

Crafting the right prompt is crucial to getting the most out of the LearningLemur AI for math tool. Think of it as briefing a colleague or delegating to a student teacher. Be clear, be specific, and include the following:

Specify the exact math topic you want to assess in your students. For example, indicate whether you want exercises on fractions or polynomials.
Define the educational level or age of your students to tailor the difficulty. Questions should be accessible yet challenging, matching the knowledge and skills of your learners.
Add any constraints. If you have specific requirements, such as simplifying answers or using decimals only, be sure to include them. This ensures the generated questions meet your pedagogical or exam criteria.
Optionally, use word problems to add context and generate relatable scenarios. Adding context or real-life situations makes problems more engaging and easier to understand for students. For instance, framing a problem in the context of shopping, travel or science helps connect math to everyday life and increases motivation.

Example Prompts

“Create 6 word problems on solving linear equations for Grade 9. Include at least one with negative coefficients and require students to simplify x.”

“Create 10 questions on simplifying algebraic expressions for 11-year-old students. Include at least one question involving the distributive property and another with factoring.”

“Create 5 questions for first-year university math students on calculating the rank of a matrix using elementary row operations.”

Use Cases in the Real Classroom

The LearningLemur math question generator adapts across levels and contexts:

For daily practice, generate variations to reinforce a concept.
For formative assessment, tailor difficulty and format.
For homework, instantly provide differentiated sets by level.
For exam prep, create question banks by topic or skill.

And since you can edit anything, it integrates seamlessly into any teaching style.

Diverse Learning Needs: Easily Tailor Content to Students’ Needs

From the teachers’ perspective, LearningLemur offers great flexibility, as educators with limited time or resources in creating math problems can now generate high-quality content more quickly. These advantages are particularly evident for teachers managing large classes, as they can personalize content while reducing preparation time.

Additionally, LearningLemur enables teachers to address the diverse needs of their students by creating customized quizzes and exercises tailored to different skill levels and learning requirements. Teachers can also assign targeted homework tasks, allowing students to strengthen specific competencies where they may need additional support.

Empowering Teachers Through Intelligent Automation

AI for math isn’t about replacing educators: it’s about empowering them. LearningLemur is a clear example of how AI question generators can reduce friction in the creative process while leaving full pedagogical control in teachers’ hands.

Whether you’re battling writer’s block or trying to generate multiple versions of a quiz quickly, this tool gives you a solid head start.

Try the AI of LearningLemur now

Uncategorized

The Role of Technology in Modern Mathematics Education

Post author By Aida Alsina
Post date June 12, 2025

Technology is no longer a guest in the classroom: it’s become a core part of the educational experience. And in the world of mathematics, it’s proving to be a powerful ally. From interactive whiteboards to adaptive AI systems, technology in math education is reshaping the way students learn and how teachers teach.

But what are the tools driving this transformation? And how can enterprises and institutions make the most of them while navigating the inevitable challenges?

In this article, we break down the evolution of digital learning tools, explore their practical impact, and look ahead to where educational technology trends are taking us.

How Technology Has Evolved Math Education

There was a time when learning math meant pencil, paper, and a lot of chalk. Today, that equation includes dynamic software, mobile apps, and real-time collaboration platforms. Over the years, technology has evolved from a classroom supplement to a strategic cornerstone of math education.

What changed? The tools got smarter, more intuitive, and better aligned with pedagogical goals. Technology in math education now means:

Receiving instant feedback and adaptive assessments.
Engaging in problem-solving through interactive scenarios.
Collaborating beyond borders with cloud-based platforms.
Visualizing abstract concepts through simulations.
Helping students to reduce math anxiety.

Digital tools for math teaching are not only enhancing understanding but also making math more approachable, flexible, and inclusive.

Key Technologies Enhancing Math Learning

From immersive 3D platforms to algebra systems that respond in real time, the toolbox for math educators is broader and more innovative than ever. Let’s look at some of the most impactful digital learning tools reshaping classrooms today.

AI and adaptive learning

Artificial intelligence is reshaping math education by enabling more personalized and adaptive learning experiences. By analyzing individual performance, adaptive systems deliver tailored exercises, offer real-time hints, and adjust difficulty levels on the fly. Many platforms now include AI-driven features to support this responsiveness.

A clear example is MathType handwriting, a tool that uses artificial intelligence to convert handwritten expressions into clean, digital equations ready to be used in your documents. These innovations represent a step forward in simplifying the transition from analog input to digital output and supporting more intuitive interaction with mathematical content in digital learning environments.

Graphing calculators and CAS tools

Graphing calculators and Computer Algebra Systems (CAS) allow students to explore equations, functions, and transformations in real time. These solutions shift the focus from mechanical calculation to analytical insight. A prime example is CalcMe, Wiris’ JavaScript-based CAS, which enables users to perform complex calculations and visualize mathematical concepts interactively across various devices.

Interactive software

Visual and interactive platforms offer higher engagement. Programs like WirisQuizzes and Learning Lemur enable learners to build, manipulate, and visualize mathematical models.

With MathType, creating complex equations becomes intuitive, helping students and teachers focus on the math, not the formatting.

LMS integration

Platforms such as Moodle and Canvas have become exponentially more powerful with integrated digital math tools. Embedding the previously mentioned platforms within these systems turns traditional coursework into interactive, dynamic experiences that meet learners where they are.

Online collaborative platforms

Math isn’t always a solo activity. Digital platforms make it easy for students to co-create solutions, share ideas, and solve real-world problems together, whether in the classroom or worldwide. Working in teams, sharing solutions, and learning from peers isn’t just good practice; it’s real-world preparation.

Challenges on the Digital Road

Of course, no transition comes without friction. Schools and universities face several challenges in adopting digital tools for math teaching:

Access inequality: Not every student has reliable internet or a personal device.
Teacher training gaps: Tools are only as effective as those using them.
Overreliance risk: Tech should enhance, not replace, mathematical reasoning.
Privacy concerns: Data protection must be part of the planning.
Maintenance and cost: Edtech isn’t a one-time purchase; it’s an evolving ecosystem.

These issues are real, but not insurmountable. They call for thoughtful planning, continuous support, and strategic investment.

Smart Implementation: Making the Most of Digital Tools

What does it take to ensure technology truly enhances math education? How can enterprises and institutions deploy digital learning tools effectively while avoiding common pitfalls?

Start with clear goals. Tech without purpose is just noise. Define your outcomes first, then choose solutions that help achieve them.
Empower teachers and invest in training. Teachers need to feel confident, not just compliant, when using digital tools for math teaching.
Ensure access, make inclusion a priority. Equitable access to devices and software should be foundational, not optional.
Blend old and new. Combine traditional instruction with digital methods. Balance is key: let tech support, not replace, core learning.
Safeguard data. Choose platforms with robust privacy protocols. Make sure every tool complies with education data standards.
Encourage exploration. Let students use technology to tinker, test, and create. Math becomes more meaningful when they take ownership of their learning.

Looking Ahead: What’s Next in EdTech for Math?

The pace of innovation is only accelerating. These educational technology trends are already reshaping what’s possible:

Smarter AI: Learning systems that adapt in real time based on student engagement.
Blockchain records: Tamper-proof credentials that simplify academic verification.
Game-based curricula: Turning math practice into immersive, story-driven experiences.
Immersive AR/VR: Math will move off the page and into the student’s physical space, making abstract ideas concrete.
Global classrooms: Seamless, multilingual collaboration across borders.
Modular microlearning: Bite-sized lessons for modern attention spans.
Accessibility: Digital tools are increasingly designed to support learners with disabilities or diverse learning needs, including visual impairments, dyslexia, and motor challenges.

How Wiris Is Leading the Change

At Wiris, we believe technology in math education should empower without overwhelming. That’s why we’ve built solutions that integrate seamlessly into classrooms while amplifying learning outcomes.

WirisQuizzes: create auto-graded, math-rich assessments inside your LMS.
MathType: author complex math expressions with ease, across any device.
Learning Lemur: generate math content and adapt the learning process to individual student needs.
CalcMe: perform complex calculations and visualize mathematical concepts interactively across various devices.

Whether you’re teaching derivatives or designing STEM assessments, Wiris helps educators turn digital potential into real progress.

Final Thoughts

The classroom is changing, students are changing, technology is changing, and math education must evolve to meet the moment.

By thoughtfully and strategically integrating digital learning tools, schools can unlock deeper understanding, greater engagement, and lifelong skills. At Wiris, we’re proud to help educators worldwide navigate this journey.

Ready to bring your math instruction into the future? Discover how Wiris can help you lead the way with cutting-edge educational technology.

Learn More About Wiris Solutions

Uncategorized

How to Calculate the Area Between Two Curves?

Post author By Aida Alsina
Post date June 5, 2025

Step-by-step Solution to an Oxford Access Exam Problem.

The Oxford University access exams are famous for their difficulty and for challenging students with mathematical problems of high complexity. In this article, we will focus on a 2020 exam problem that involves calculating the area between two curves. Through a clear and detailed analysis, we will break down each step necessary to approach and solve this mathematical challenge.

If you are interested, feel free to also visit our other article, “How to solve an equation of degree 16”, where we explore another challenging Oxford exam problem.

The problem statement is as follows:

A line is tangent to the parabola y=x² at the point (a,a²), where a>0.

The area of the region bounded by the parabola, the tangent line, and the x-axis equals

(a) a²⁄₃, (b) 2a²⁄₃, (c) a³⁄₁₂, (d) 5a³⁄₆, (a) a⁴⁄₁₀

This is a classic calculus problem that, as we will see, requires the use of both derivatives and integrals. Don’t worry; we’ll break it down into three clear and simple steps. Let’s get to it!

How to Find the Equation of the Tangent Line?

Before starting with the calculations, let’s visualize the problem. Below, you’ll see a graph illustrating the parabola y=x² and the tangent line at the point (a, a²), where a>0. In this graph, we can see how the tangent line touches the curve of the parabola at a single point without crossing it.

Now, to proceed, we first need to find the equation of the tangent line at that specific point. Since the tangent touches the parabola at point (a, a²), we need to determine both its slope and its equation.

This is where the derivatives come into play. The slope of the tangent at any point on the parabola is given by the derivative of the function y=x². We start by calculating the derivative: dy/dx=2x

This means that the slope of the tangent at any point on the parabola is 2x. To find the slope at the tangent point (a, a²), we substitute in the derivative:

Therefore, the slope of the tangent line at point (a, a²) is 2a.

Now that we have the slope, we can write the equation of the tangent line using the formula for the line in its form:

y – y₀ = m (x – x₀)

Where m is the slope of the line, and (x₀ , y₀) is the point through which the line passes. In our case, the point of tangency is (a, a²), and the slope is 2a, so we substitute these values in the formula for the line:

y – a² = 2a (x-a)

y – a² = 2ax – 2a²

y = 2ax – a²

So, the equation of the tangent line at the point (a, a²) is:

y = 2ax – a²

Find the Intersection Points

Now that we have the equation of the tangent, we need to find the points of intersection between this tangent and the x-axis, that is, when y=0. This will allow us to determine the limits of the region whose area we want to calculate.

The area to be calculated is marked in orange in the graph below.

Substituting y=0 in the tangent equation and solving for x we obtain:

0= 2ax – a²

2ax = a²

x = a/2

So the tangent cuts the x-axis at point

P=(a/2, 0 )

Calculate the Area Between the Parabola, the Tangent and the X-axis

Once we have the equation of the tangent and we know the points of intersection, we can proceed to calculate the area of the region bounded by the parabola, the tangent and the x-axis. For this, we use the definite integral, which allows us to find the area between two curves.

The area we want to calculate is obtained by subtracting two regions. In the first graph, you can see the total region we are interested in. However, part of this area is specifically delimited in the second graph, which we must subtract to be left with only the part we are looking for.

Let us calculate both integrals:

a) Integral of graph 1:

definite integral of x squared from 0 to a. It equals the integral from 0 to a of x squared dx, which is equal to x cubed over 3 evaluated from 0 to a, resulting in a cubed over 3.

b) Integral of graph 2:

Now that we have solved all the integrals, we subtract the results to obtain the total area:

In conclusion, the area of the region bounded by the parabola y = x², the tangent y = 2ax – a² and the x-axis, is:

a sup 3 / 12

Therefore, option c is the correct answer

And so we come to the end of this fascinating problem! If you enjoyed this analysis or found it useful to better understand the solving process, feel free to share it with other math enthusiasts!

Now we challenge you: do you dare to calculate the area formed by the curve y = x³ and its tangent at the point (1,1)? Share your solution or your ideas in the comments. We’d love to hear your approach!

Try WirisQuizzes to generate this exercise or others!

Uncategorized

Towers of Hanoi: A Math and Programming Challenge

Post author By Aida Alsina
Post date May 21, 2025

Towers of Hanoi: A Math and Programming Challenge

The Towers of Hanoi is one of the most iconic puzzles in the field of mathematics and computer science. With its simple structure and transparent rules, this problem has captivated mathematicians and amateurs alike, making it an intriguing challenge since its creation.

According to the legend, the Towers of Hanoi were conceived by a group of monks in an ancient temple in India. In the center of this temple, three golden pillars stood, upon which rested 64 disks of various sizes, arranged in the first pillar in an orderly fashion: the largest disk at the base, with progressively smaller disks stacked on top, until reaching the smallest disk, placed at the top.

The monks’ objective was to move all the disks from the first pillar to the third, using the central pillar as an auxiliary. To accomplish this task, they had to follow a strict set of rules:

Only one disk can be moved at a time.
A disc can never be placed on top of a smaller disc.

Legend says that when all the disks were correctly transferred to the last pillar, the world would come to an end. However, how close is the end of the world, really? How long would it take to complete this challenge?

This challenge was officially introduced by the French mathematician Édouard Lucas in 1883 as part of his research in number theory and quickly became a popular problem.

Next, we will analyze the solution of this problem from a mathematical perspective, breaking down its key principles and explore how it transforms into an interesting programming challenge.

Recursion as a Solution to the Puzzle

The challenge of the Towers of Hanoi is not only in moving the disks from one pillar to another following the strict rules but also in calculating how many moves are needed to solve the problem of moving n disks from the origin pillar to the destination pillar.

The most efficient way to approach this problem is through recursion, a fundamental technique in programming. Recursion allows the problem to be divided into smaller subproblems, where the solution of each subproblem leads to the solution of the original problem.

In the case of the Towers of Hanoi, recursion applies naturally, and we can divide the problem of moving n disks into three steps:

Move the first n – 1 disks from the origin pillar to the auxiliary pillar, using the destination pillar as the auxiliary.
Move the largest disc (the disc n) from the origin pillar to the destination pillar.
Move the n – 1 disks from the auxiliary pillar to the destination pillar, using the origin pillar as an auxiliary.

We can repeat this pattern recursively, reducing the number of discs with every step until we reach a base case where only one disk remains, which we just directly move.

In mathematical terms, the minimum number of moves to solve the problem with n disks equals the number of moves needed to solve it for n – 1 disks, plus one to move the largest disk, plus the number of moves needed to solve it for n – 1 disks again. This formula yields the result:

TH( n ) = TH( n – 1 ) + 1 + TH( n – 1 ) = 2TH( n – 1 ) + 1

Where TH( n ) represents the number of moves needed to solve the problem with n disks.

From the above formula, we will substitute each TH( i ) by its corresponding expression until we reach TH( 1 ) . Then, we will replace TH( 1 ) by 1, since, with only one disc, it is possible to move it directly to the destination pillar, which justifies that the minimum number of moves in this case is 1.

Let’s see the expression developed:

We first substitute TH( n – 1 ), and we obtain:

TH( n ) = 2TH( n – 1 ) + 1

TH( n ) = 2( 2TH( n – 2 ) + 1 ) + 1 = 2²TH( n – 2 ) + 3

Now we substitute TH( n – 2 ):

TH( n ) = 2²TH( n – 2 ) + 3

TH( n ) = 2²( 2TH( n – 3 ) + 1 ) + 3 = 2³TH( n – 3 ) + 7

If we continue substituting in this way, we will arrive at a general formula:

TH( n ) = 2^k TH( n – k ) + (2^k – 1)

Where k is the number of steps backward in the recursion.

For k = n – 1 we arrive at TH( 1 ), the base case:

TH( n ) = 2²TH( n – 2 ) + 3

We know that TH( 1 ) = 1, then we obtain:

TH( n ) = 2^{n – 1} · 1 + ( 2^{n – 1} – 1 ) = 2ⁿ – 1

We have shown that the number of moves needed to solve the Towers of Hanoi with n disks is:

TH( n ) = 2ⁿ – 1

Solving the Puzzle Using Mathematical Induction

Another way to approach the solution to the Towers of Hanoi puzzle is to use mathematical induction, a technique used to prove that a statement is true for all natural numbers, based on two fundamental steps: the base case and the inductive step.

In this case, the goal is to show that to move disks from the first pillar to the third pillar, 2ⁿ – 1 moves are required. Let’s break this process down with a demonstration by induction.

Base case:

Suppose we have only one disk. In this scenario, it is clear that only one move is needed to move the disk from the source abutment to the target abutment. That is, for n = 1, the number of moves required is 2¹ – 1 = 1, which is true.

Inductive step:

Now, suppose that the statement is true for n = k, i.e., that to move k disks requires 2^k – 1 moves. What we must prove is that the statement is also true for n = k + 1.

To move k + 1 disks, we first need to move the upper disks from the origin pillar to the auxiliary pillar, using the destination pillar as an auxiliary. According to our inductive hypothesis, this will take 2^k – 1 moves.

Next, we move the larger disk (the disk k + 1) from the origin pillar to the destination pillar, which requires just 1 move.

Finally, we move the k disks from the auxiliary pillar to the destination pillar, using the origin pillar as an auxiliary, which will also take 2^k – 1 moves, according to our inductive hypothesis.

Therefore, the total number of moves required to move k + 1 disks is:

(2^k – 1) + 1 + (2^k – 1) = 2^k+1 – 1

Thus, we have shown that if the statement is true for n = k, it is also true for n = k + 1.

As we have observed, it is true for 1. By mathematical induction, we can conclude that for n disks, the minimum number of necessary moves is 2ⁿ – 1.

Going back to the legend, if the monks were extremely fast and could move a disk in just one second, we could calculate how long it would take to complete the challenge with 64 disks. According to the formula, the total number of moves would be 2⁶⁴ – 1, approximately 18.4 quintillion moves. With each of these taking just 1 second, this gives us 18.4 quintillion seconds. Converting these into years, we get that the total time would be approximately 581.4 billion years. So, if the legend turned out to be true, the end of the world would still be a long way away!

CalcMe formula We note that the function describing the number of moves needed to solve the Towers of Hanoi is exponential, meaning that it grows rapidly as we increase the number of disks. In the following graph, we can visually see how this function spikes, clearly illustrating the exponential growth.

graphic formula

This visual support allows us to confirm, once again, that completing the challenge with 64 discs would take an extraordinary amount of time.

If you liked this mathematical challenge and you were surprised by its solution, don’t hesitate to share this article! And if you dare, test your skills by solving the Towers of Hanoi puzzle: will you manage to move the disks in the exact number of steps? Let us know your experience!

Uncategorized

AI in Mathematics: The Revolution in Scientific Writing

Post author By Aida Alsina
Post date May 6, 2025

Artificial Intelligence (AI) is a field of computer science that seeks to develop systems able to perform tasks that traditionally require human intelligence, such as logical reasoning, problem solving, and learning. Based on advanced algorithms and machine learning models, AI can analyze large volumes of data, identify complex patterns and adapt autonomously to new contexts.

In mathematics, these capabilities are transforming the way knowledge is generated, verified, and communicated. From automating demonstrations to generating detailed explanations, AI is facilitating the work of researchers, teachers, and students. In addition, its ability to process natural language is improving the writing of mathematical papers, making texts more accessible, accurate and structured.

In this article, we will explore how AI in math is revolutionizing mathematical writing and how Wiris’ tools are part of this advancement.

The Role of AI in math and the Creation and Editing of Mathematical Content

Writing scientific papers in mathematics has always been a challenge due to the complexity of their notations and symbols. Traditionally, mathematicians have relied on handwriting or the use of complex typesetting languages. However, with the advent of AI, the process of creating and editing scientific documents has evolved exponentially.

Artificial intelligence has brought multiple benefits to the writing of mathematical papers, facilitating both the writing and the editing and publication of academic papers. Below are some of the main ways in which AI is impacting this area.

Handwriting recognition

One of the most significant advances has been the development of systems capable of interpreting and digitizing handwritten notes. Traditionally, mathematicians wrote their equations and proofs on paper, which was time-consuming if they needed to transcribe them into a digital format. With AI-based handwriting recognition tools, such as those built into MathType, it is now possible to directly handwrite equations into our touch devices and automatically convert them into editable digital text.

This not only saves time but also reduces transcription errors and allows you to work more efficiently. In addition, this type of technology is especially useful for students and teachers, as it facilitates the conversion of notes into organized documents without the need for manual typing.

Formula automation

In the creation of mathematical articles, writing formulas accurately is essential. Traditionally, this required specialized tools that, while powerful, could be complex and demanded a deep understanding of their syntax and structure, such as LaTex.

Advancements in technology have enabled the development of tools like MathType, which simplify equation writing without the need for coding. Instead of memorizing commands and code structures, users can simply enter their formulas through an intuitive graphical interface. This democratizes access to mathematical writing, allowing more people, regardless of their technical expertise, to create high-quality mathematical documents.

Another key aspect is the automatic correction of errors in equations. AI in math can identify inconsistencies in formula writing and suggest real-time corrections, preventing mistakes that could compromise the validity of an academic paper.

Optimization of editing

Another benefit that AI has brought is the optimization of the editing of mathematical documents. In the past, mathematicians and scientists had to manually check their documents for errors in equations, notation and text structure. Today, there are AI algorithms embedded in editing programs that can analyze the consistency of mathematical expressions, suggest improvements in writing and detect inconsistencies.

AI-based writing assistants, such as those integrated into advanced text processors, can help improve the clarity and readability of mathematical articles. In addition, they provide stylistic recommendations to give the paper a logical and easy-to-follow structure, which is crucial in academic paper writing.

The integration of AI into mathematical writing is not just an evolution but a revolution that is transforming the way we create, edit, and share scientific knowledge. Tools like Wiris, with their powerful capabilities, are making mathematical writing more accessible, efficient, and error-free. As these technologies continue to advance, they will further bridge the gap between human intuition and machine accuracy, making the work of researchers, teachers, and students alike easier. If you found this exploration interesting, share it with your peers and join the conversation about the future of AI in math.

If you’d like to find out more about our products, please feel free to get in touch with our Sales team at sales@wiris.com for more information.

Uncategorized

Wiris in the Classroom: Learn and Practice with Unlimited Exercises

Post author By Aida Alsina
Post date April 16, 2025

Learning mathematics requires more than just understanding theories: the key to mastering complex concepts and honing skills lies in continuous practice. However, traditional methods often limit the learning process: once the student has solved a problem, the challenge disappears, and exams often repeat the same questions, thus losing their value as an assessment tool. Imagine having the possibility to generate unlimited exercises with random variables, adapted to the needs of each student. With Wiris, this is now possible.

In addition, it has an automatic correction functionality which means that while the exercise is being generated, the answer is calculated simultaneously. This allows instant feedback, making it easier to learn and correct mistakes immediately.
For teachers, this tool offers a key advantage: the possibility of generating an infinite number of exercises, with each one being unique. In this way, teachers can create varied and personalized learning experiences, ensuring that students face new challenges every time they practice.

For their part, students enjoy constant practice which allows them to reinforce their skills without the worry of repeating the same exercises. In addition, this ability to generate unlimited exercises gives them excellent preparation for exams.

Practical example: Solving a system of linear equations

To illustrate how this tool works and how it can be used in the classroom, we will focus on solving systems of linear equations. We chose these types of problems because they represent a common mathematical challenge faced by all students and offer the possibility to explore different problem-solving methods. In addition, systems of equations can have various characteristics, such as being compatible, incompatible, or indeterminate, allowing students to work with a wide range of situations.

Let us imagine that we have the following system of linear equations with three unknowns:

We are going to solve it using Gauss’s Method. The first step is to write the system in the form of an augmented matrix:

Now, we will apply elementary operations to reduce the matrix to its row echelon form.

Therefore, we obtain:

From equation 3 of the system, we find the variable z:

Unlimited generation of systems of linear equations with random variables

Now that we have solved this system, for further practice, we would like to be able to generate new systems of equations of the same form but with random values.

These systems would take the following form:

Below, we will show you a code example to implement this functionality and generate given random systems of equations. With Wiris, it is totally possible!

r() := random(-4,4)

sol = [r() with i in 1..3]

[a,b,c] = sol

repeat

A = [[r() with i in 1..3] with j in 1..3]

until determinant (A) != 0

b = A * sol

ec = {}

for i in 1..3 do

ecaux = A.i * [x,y,z]

ec = append(ec, ecaux = b.i)

end

{ec1, ec2, ec3} = ec

r() := random(-4,4): This function generates a random number between -4 and 4.

sol = [r() with i in 1..3]: Here, three random values are generated that will represent the solutions of the system of equations.

[a,b,c] = sol: The generated values are assigned to the unknowns of the system.

repeat…until determinant(A) != 0: This block ensures that the coefficient matrix A is invertible (i.e., its determinant is not zero), which guarantees the system has a unique solution.

b = A * sol: The result vector b is calculated by multiplying the coefficient matrix A by the solutions sol

for i in 1..3 do: In this cycle, the three equations are generated, using each of the rows of the matrix A and the solutions sol.

{ec1, ec2, ec3} = ec: Finally, the three generated equations are stored in the variables ec1, ec2, and ec3, ready to be used in new exercises.

Using WirisQuizzes and implementing this code as a question, we can obtain the following results:

Reloading another system with the symbol “=” generates a new set of equations, as shown below:

For more details, you can consult the complete product documentation here.

If you have found this article useful, we would love you to share it with other colleagues, teachers or students. We would also be delighted to hear your opinion and any suggestions you may have.

Check Out WirisQuizzes

Uncategorized

What is the Birthday Paradox?

Post author By Aida Alsina
Post date April 8, 2025

The Fascinating Probability of Sharing a Date

The birthday paradox is a mathematical concept that invites us to challenge our intuition. A paradox, in essence, is a statement or result that, although seemingly contradictory or contrary to common logic, proves to be true when analyzed closely. In the case of the birthday paradox, the scenario is simple but puzzling: how many people must be in a room for the probability of at least two sharing the same birthday to be greater than 50%?

The result will be surprisingly low, but do you dare to answer before you continue reading to reveal the answer?

The most intuitive way to approach the paradox would be to think that it takes at least 183 people, half the days of the year, for the probability of two people sharing the same birthday to be greater than 50%. However, the reality is very different.

To understand how this probability is calculated, let us recall that the basic formula of any probability consists of dividing the favorable cases by the total cases.

In this case, rather than calculating the probability of a coincidence directly, it is easier to first calculate the probability that no two people share a birthday. After determining this probability, subtracting it from 1 (that is, calculating the complement) gives the probability that at least two people share a birthday.

To calculate the probability that no two people share a birthday, we will proceed constructively, evaluating scenario by scenario.

If there is only one person in a room, there is no chance that they will share their birthday with someone else since no other people are present. Therefore, the probability that their birthday is unique is 100%, i.e., 365/365.
Now, if a second person comes in, the probability that their birthday will be different from the first person’s is 364/365, since there are only 364 days remaining in which they can be born to avoid a coincidence.
If a third person is added, the probability that their birthday does not match anyone else’s is 363/365 since there are now two busy days.

Therefore, for n people, all the above conditions must be met simultaneously: that the second person does not share a birthday with the first, that the third person does not share a birthday with the previous two, and so on. This implies that the probability that none of the n people share the same birthday is the product of the individual probabilities:

And finally, we obtain:

Analyzing probabilities for different values of n

Let us examine the results for different values of n:

For n=10 we obtain that P (at least one coincidence) = 11,61%

CalcMe representation of the equation

This calculation has been created using CalcMe

For n=15 we obtain that P (at least one coincidence) = 25,03%
For n=23 we obtain that P (at least one coincidence) = 50,05%
For n=50 we obtain that P (at least one coincidence) = 96,53%
For n=60 we obtain that P (at least one coincidence) = 99,22%

As we can see from the examples above, to achieve a probability of more than 50%, only 23 people in the same room are needed. This result may seem surprising, but it makes sense if we consider that with 23 people, 253 different pairs can be formed, and each of these pairs represents a chance for two people to share the same birthday. Even more surprising is that to have a probability greater than 99%, only 60 people are needed, demonstrating how the number of possible combinations grows rapidly with every new person added.

This is the graph of the probability distribution:

Graph showing the probability of two people sharing a birthday, with the number of people on the X-axis and the probability on the Y-axis, expressed as a percentage.

In contrast, if instead of calculating the probability of coincidence between any pair of people we consider the probability that someone in a room of n people (excluding you) specifically shares your birthday, the calculation is different. This probability is given by:

For n = 22, this probability is about 0.059 (5.9%), which is quite low. In fact, there would need to be at least 253 people in the room for this probability to exceed 50%.

The birthday paradox is a fascinating reminder of how our intuition can fail when confronted with mathematical logic.

Now that you know the birthday paradox and its key concepts, can you calculate the probability that exactly k people within a group of n people share their birthday? We’d love to hear your results in the blog comments! If this article surprised you, share it with your friends so that they can learn more about this mathematical paradox. What’s more, if you’re interested in trying these calculations for yourself, have a look at CalcMe.

Try CalcMe